How Calculus in 5 Hours Improves Your Understanding of Calculus and Gives You the Confidence You Need
Listen, many popular textbooks reach a whopping 1,000 pages to introduce Calculus, needlessly frustrating and overwhelming students.
GET IT NOW
But too many details can impair learning - especially when you're learning something for the first time - creating doubt and uncertainty in your ability to understand. What you need is a straightforward guide to give you the basic concepts.
Fortunately, the book Calculus in 5 Hours is focused on you and what you need to do well. Here's what it will do for you:
- Organize your understanding of Calculus for quick and easy recall on tests and homework assignments
- Present straightforward drawings that demonstrate concepts with minimal effort on your part
- Highlight simple examples without burdening you with useless details
Focus on what's truly important to gain knowledge and achievement as fast as possible with less effort.
Get Calculus in 5 Hours now for $2.99
Want to know how this helps beginning Calculus students?
Learning is about organizing the neurons in your brain. When you first learn a subject, your neurons are disorganized and randomly arranged. This makes it difficult to remember concepts and ideas quickly.
But as your learning increases, those neurons form chunks of information that are easily accessible. Which means you can recall them quickly and effortlessly for tests and homework assignments.
For example, think about the letters C, A, L, C, U, L, U, and S. You're familiar with the alphabet, but your mind has put them together to form the word CALCULUS which has a separate meaning from the individual letters. You don't think about the alphabet because you've put meaning into the word.
That's chunking.
By the way, an excellent resource for learning about chunking and its application to math is Barbara Oakley's book, A Mind For Numbers: How to Excel at Math and Science (Even If You Flunked Algebra).
According to Barbara Oakley, one of the first steps in learning a subject is to "create conceptual chunks, mental leaps that unite scattered bits of information through meaning."[1]
Let's say you wanted to learn geography. You could start by memorizing every city on the planet, but that would be stupid. Instead, a smart method would be to start with the big chunks. Learn the seven continents and where they are on a map. Then get a sense of the biggest countries in each continent and something about each one. Then move to smaller countries and maybe some important cities like the capitals.
By doing it this way you'll learn more at a faster pace . . .
In Calculus in 5 Hours you're not given every tiny bit of information about Calculus like most textbooks give you. You're exposed to only the major chunks of information needed in a first semester Calculus course in college.
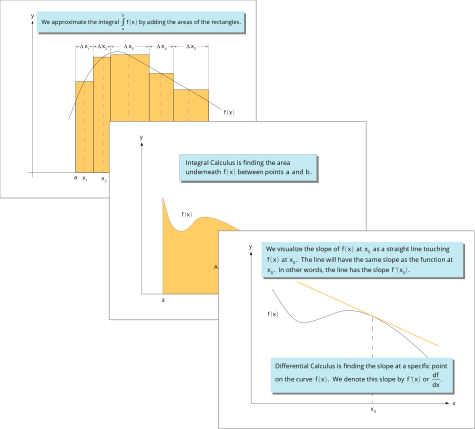
For example, Calculus can be broken down into two major chunks:
- Differential Calculus
- Integral Calculus
Differential Calculus is how steep a function is. It can be very steep like a tall mountain or not very steep at all like a small hill. Integral Calculus is about the amount of area under a curve.
Calculus in 5 Hours gives you a step-by-step process for learning Calculus quickly without overwhelming you with too many details. This is done with simple pictures and clearly defined rules so you don't have to wonder what you really need to know. It's all laid out for you.
To make learning easier, Calculus in 5 Hours breaks everything down into 5 parts or major chunks:
Functions and an Overview of Calculus (27 minutes)
- The 4 characteristics of every function so you won't be confused by the notation in your assigned textbook. Page 13.
- How areas are related to integrals and the only notation you'll ever need to describe them. Page 30.
- What a derivative is and the two notations for a derivative used in every Calculus book. Page 29.
- The 2 core concepts of Calculus explained in less than 4 pages and with only 3 pictures. Page 28.
- How to find the leading coefficient and degree of any polynomial. Page 24.
- The 5 ways to combine two functions you must know. Page 25.
- The two characteristics of every straight line. Page 22.
Derivatives (127 minutes)
- A step-by-step recipe for applying the Chain Rule, so problems that take hours of work can be solved in one line. Page 53.
- How functions are used to describe the real world and how to make sure formulas can model reality. Page 55.
- How to calculate average and instantaneous rates of change . . . and what each of them means. Page 60.
- The second derivative - how to calculate it and 4 simple pictures to give you its meaning. Page 64.
- The real-world meaning of derivatives that you're guaranteed to be tested on. Page 59.
- The 4 most important rules for derivatives you will be tested on. Page 38.
- How to find the approximate value of a function using only derivatives and a Tangent Line. Page 44.
- How to user the Power Rule to find the derivative of a polynomial. Page 45.
- A crazy but effective technique for memorizing the Quotient Rule. Page 50.
- The simple relationship between function limits and derivatives. Page 35.
- The easy-to-memorize formula for the slope of a line. Page 33.
- The Product Rule in both Liebniz and prime notation. Page 47.
- The difference between secant and tangent lines. Page 32.
- The formula for the Tangent Line. Page 43.
The Geometry of Functions (67 minutes)
- Maxima and minima easily explained in one sentence and a picture. Page 86.
- Increasing and decreasing functions explained in 2 freakishly simple drawings. Page 74.
- How derivatives are used to find where a function is increasing or decreasing. Page 82.
- Inflection points and why you can't find them with the naked eye. Page 100.
- Concavity and how you can determine it with second derivatives. Page 98.
- What a critical point is and why it makes finding maxima and minima easy.
- The 4 straightforward steps for finding maxima and minima. Page 94.
- The Mean-Value Theorem explained in one picture. Page 72.
One area very well treated is "Geometry of Functions" as it relates to critical points, inflections, concavity and second-derivative tests.

Integrals (67 minutes)
- Where the crazy integral notation comes from and why knowing this will help you remember them. Page 105.
- The Fundamental Theorem of Integral Calculus and why it makes solving integrals trivial. Page 114.
- A simple, 2-line table explaining the difference between definite and indefinite integrals. Page 106.
- Why you can safely ignore the constant of integration in every definite integral. Page 115.
- How to add combinations of integrals together so you can solve problems faster. Page 118.
- The 5 rules of antiderivatives you'll have to know to get an A+ in your class. Page 110.
- How integrals are related to the areas of rectangles. page 104.
- The 2 ways an integral can be negative. Page 121.
- How to find averages with integrals. Page 125.
Exponential and Logarithm Functions (12 minutes)
- The domain, range, and graph of the exponential and logarithm functions so you know exactly what they are. page 128.
- The properties of exponential and logarithm functions you must memorize. Page 131.
- The definition of a logarithm and why it's much easier than you think. Page 130.
- The integral of 1/x and how it's related to the logarithm function. Page 134.
- How exponential and logarithm functions are related to each other. Page 130.
- The derivative of the exponential and logarithm functions. Page 133.
So that's how . . .
Calculus in 5 Hours organizes the information for easy learning and quick recall so you don't have to. But there's several more things you need to know about the book.
Every chapter is broken down by the number of minutes so you can plan how long you need to study. The average chapter size is 3.4 pages. Ideally, you'll want to go through the book quickly so you get all the major concepts. Then use the book as a reference knowing you won't have to spend hours looking for what you need to know.
Calculus in 5 Hours uses pictures (instead of confusing math notation) whenever it can. This means you'll learn faster and remember the concepts easier. Every picture is available as a large, full-color image and available through this website. And if you have a black and white version of the book, it points you to the exact page where you can view and download all the images. And there's a list of all the figures in the back of the book, so you can find them easily when you want to remember a concept.
The book provides crisp, clear examples of graphs of all the functions, derivatives and integrals discussed, as well as a welcome glossary of terms such as "function," "derivative" and "integral. It also offers call-out boxes with important concepts outlined.

Unlike most mathematics textbooks, Calculus in 5 Hours is specially designed for Kindle and Apple devices. That means the formulas are crisp, clear, and zoomable for easy reading.
And there's a glossary in the back defining the important concepts and words. It points to the chapter where the concept is introduced so you can find out more. This is perfect for a quick review or when you're trying to remember something important.
Finally, every exercise has a link to its solution accessible on this website. And several exercises have interactive calculators for exploring the concepts on your own. (Or for doing homework assignments quickly and easily.)
Those who know Calculus will succeed no matter what degree or occupation they choose.

Today there's a big push to emphasize STEM (science, technology, engineering, and math) skills. That's because these skills are in big demand and important for succeeding in fields that are not traditional STEM fields.
For example, some law programs require Calculus. And statistics, which is a required subject for many fields including psychology, is based entirely on Calculus.
In other words, Calculus is everywhere. It's one of the few subjects that almost every quantitative and qualitative college degree has in common. You simply can't avoid knowing it.
That's why three whole chapters are spent teaching you simple, real-world applications of Calculus.
How much is all this Calculus knowledge worth - especially when it's applicable to almost every high paying college degree you can get?
And how much more employable will you be when knowing Calculus leads to higher grades in all your other classes?
Finding the right resource is important. Too many students spin their wheels with complicated textbooks that throw every detail at them - even if it's not needed.
Sometimes all you need is having something said the right way. Or a picture that brings it all together. Or a step-by-step process. Or a guide that lays it all out for you. Anything that makes the subject clearer.
This easy reading self-help book distills complex mathematical concepts into simpler, easier-to-digest bullet points for the mathematically challenged or for those needing a quick review of the subject.

The time you can save learning Calculus faster than every one else in your class can give you more time to study and make better grades. Or more time to live the life you want.
Georgetown University estimated that the average lifetime earnings of a Bachelor's degree holder was $2.7 million. For those without a college degree it's 75% lower.[4]
Today, the average price of a beer in a bar in New York city is $8.97.[3]
The average cost of a Big Mac is $3.99.[2]
Calculus in 5 Hours is $2.99.
Is it worth spending $2.99 to make your life easier so you can learn Calculus faster? All so you can make an extra 1 million over your lifetime?
Get the one resource that will save you time as you learn this valuable subject. Calculus in 5 Hours will give you the knowledge faster and with less effort on your part.
Get Calculus in 5 Hours now for $2.99
P.S. Calculus in 5 Hours is available to libraries through Overdrive, Biblioboard, and other well-known companies. If you go here, there's a PDF you can print out and give to your librarian so they have the information they need to order it.
References
- Oakley, Barbara and Terrence Sejnowski. Learning How to Learn: Powerful mental tools to help you master tough subjects. Coursera, www.coursera.org/learn/learning-how-to-learn.
- McDonald's Prices. FastFoodMenuPrices.com, www.fastfoodmenuprices.com/mcdonalds-prices. Accessed June 13, 2019.
- Beer Prices Around the World. The Wall Street Journal, graphics.wsj.com/table/BEER_062415. Accessed June 13, 2019.
- Carnevale, Anthony P., et al. The College Payoff: Education, Occupations, Lifetime Earnings. The Georgetown University Center on Education and the Workforce.


